What Is NPV?

Businesses around the globe use the net present value (NPV) to evaluate whether they should invest in a new capital project. It tells them how much money an investment will potentially bring back to the company, considering the capital the firm has spent to realize it.

In this article, we define and calculate the net present value, give reasons for discounting future cash flows, provide an elaborate example, list possible limitations of the NPV measure, and give advice on the next steps of your career journey.

Table Of Contents

• What Is the Net Present Value?
• How to Calculate the Net Present Value
• Why Are Future Cash Flows Discounted?
• The Net Present Value Formula: Example
• NPV Interpretation
• Limitations of the Net Present Value
• Showcasing NPV Calculation Skills on Your Resume
• Next Steps
• FAQs

What Is the Net Present Value?

Before all else, you need to understand the components of the net present value as individual entities. So, we’d better break the term down into two separate definitions—NET and PRESENT VALUE:

• NET represents any cash inflows and outflows that investors consider when calculating a project’s NPV.  
• PRESENT VALUE refers to today’s value of a future amount of money.

At large, the net present value of an investment is the present value of its future cash inflows minus the present value of the investment’s cash outflows. While it may sound complex in theory, NPVs are actually a big part of our daily lives, often without us even realizing it. When buying a new car, for example, you will evaluate the purchase price, loan payments, maintenance and insurance costs, and even possible resale value. These are all future cash flows that you will consider before investing in a new vehicle.

How to Calculate the Net Present Value

Here’s the NPV formula you need to use:

NPV=\sum_{t=0}^N \frac{CF_{t}}{(1+r)^t}

The term CF_t is the expected net cash flow at time t, N is the projected life of the investment, and r is the discount rate (also known as the opportunity cost of capital).

You can calculate the NVP of an investment in four easy steps:

1. Identify all inflows and outflows related to the investment.
2. Choose an appropriate discount rate, which allows for obtaining the present value of the cash flows.
3. Use the discount rate to find the present value of each cash flow. Inflows are positive and increase NPV, while outflows are negative and decrease NPV.
4. Sum up all discounted cash flows and find the net present value of the investment.

Why Are Future Cash Flows Discounted?

Are you familiar with the time value of money concept? It implies that having a dollar today is more valuable than receiving a dollar tomorrow. In other words, what we receive today has a potential earning capacity. That’s why any person would prefer to get the money sooner rather than later. Owning that one dollar in the present means you can immediately invest it to earn some interest.

The NPV formula accounts for the time value of money by discounting future cash flows. When you calculate how much your investment can earn in five years, you are projecting a profit you haven’t yet realized—and that’s a time during which a comparable amount could actually give you an additional worth of interest. To compute investment returns realistically, companies discount future cash flows. In this way, they take into account that $2 million today won’t have the same value as $2 million in five years. In other words, the value of money changes over time.

What about the risk that comes with investing? Indeed, that’s the second reason organizations discount future cash flows in the net present value calculation: to adjust for the risk of an investment. If there’s a project with a high level of risk, you’ll see that reflected in the high discount rate that goes with it. On the other hand, a relatively safe investment, such as having cash inflows from the US Treasury Bill, calls for a low discount rate.

The Net Present Value Formula: Example

Suppose that Alpha Corporation is a large company operating in the shipbuilding sector. Its R&D department has made a scientific breakthrough: they have developed a new ship prototype that will travel 20% faster than existing ship models, and it will be 10% more efficient in terms of fuel consumption. However, this innovation requires the construction of a new shipbuilding plant. The project consists of an initial investment of $150 million and will generate after-tax cash inflows of $70 million per year for the next 3 years, as shown in the following timeline:

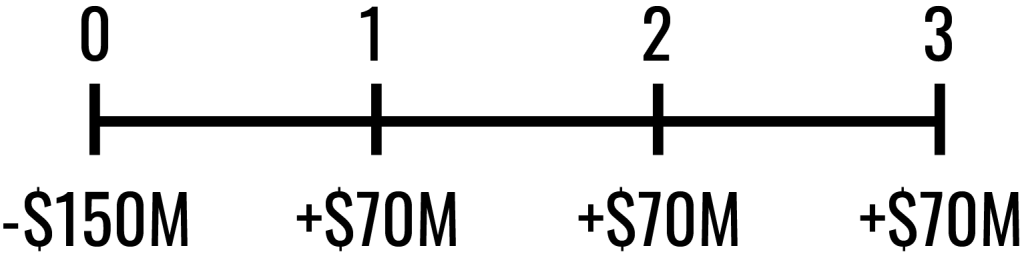
The company’s finance department has assessed that the project’s cost of capital is 9%. And the firm’s CEO wants to know whether the investment would make economic sense.

How do you find out whether this would be a profitable business decision?

You need to determine the net present value of the project. By substituting the known parameters, we obtain the following:

NPV= -\$150,000,000 + \frac{\$70,000,000{_1}}{(1+0.09)^1} + \frac{\$70,000,000{_2}}{(1+0.09)^2} + \frac{\$70,000,000{_3}}{(1+0.09)^3}= 

= -\$150,000,000 + \$64,220,183 + \$58,917,600 + \$54,052,844 = \$27,190,627

Let’s follow the four steps we’ve outlined earlier to obtain the net present value.

Step 1: The initial cost of the new plant is $150 million, which is denoted with a negative sign because this is a cash outlay. You might notice that the initial investment is not discounted at the opportunity cost of capital because it happens at time 0, which is another way to say it occurs in the present moment.

Step 2: Then, we start with the first cash inflow of $70 million, which is discounted by (1+9%) raised to the power of 1.

Step 3: Similarly, we apply the same procedure to the rest of the cash flows.

Step 4: By summing it all up, we obtain a positive value of $27,190,627.

Having a positive NPV means that Alpha Corporation can go ahead with the project because it creates value. It had the opportunity to invest in a new plant by paying $150 million for an investment worth $177.19 million.

Therefore, Alpha’s shareholders increased their wealth by $27.19 million.

Calculating NPV is easier when the number of cash flows is small. However, when we consider bigger investment projects, it is a bit more difficult to estimate. That’s why people started using financial calculators and spreadsheet software.

In practice, most professionals calculate NPV in Excel, as this saves them a lot of time and effort. Others work with a ready-to-use NPV Excel template that can be applied to various projects and is easily customizable.

NPV Interpretation

For investors, there is a clear-cut rule as to how they interpret NPV. An investment should be accepted if the net present value is positive, and rejected when it is negative:

• If NPV is higher than 0, we invest in the project;
• If NPV is lower than 0, we do not invest in the project.

When you calculate the NPV of a project, you use the opportunity cost of capital as the discount rate. And the opportunity cost is the alternative return investors forgo when they undertake an investment. When NPV is positive, the investment adds value because it compensates for more than the opportunity cost of capital. That’s why a company undertaking a positive NPV project creates value. The opposite is also valid—accepting investments that have a negative NPV isn’t financially viable.

Limitations of the Net Present Value

Based on Underlying Assumptions

When we talk about the future, there is a great level of uncertainty about the unfolding of events. Calculating NPV is no exception. Companies have no guarantee that their plans will work out, so there’s a lot of room for error. At the end of the day, estimates may prove to be wrong, and so do all financial projections that go with them. That’s why the NPV calculation is only as good as its underlying assumptions.

Possible Upward Bias

Of course, any business venture begins with the aspiration and optimism that it will be a great success. And that’s a predisposition for the people involved in the project to believe in it. They feel excited to build that new engineering plant or buy cutting-edge equipment. As a result, the enthusiasm of people estimating investment returns may sometimes affect their judgment and underlying assumptions. Because these individuals are too close to the entire project, they are prone to taking an optimistic approach to assessing future cash flows.

Highly Sensitive to the Discount Rate

One of the greatest disadvantages of the net present value has to do with its sensitivity to the discount rate which is often difficult to project with accuracy. Focusing solely on projects with a low cost of capital may not always lead to optimal results, as it eliminates lucrative business opportunities. Similarly, having an excessively high cost of capital could hinder potential good investments. In practice, it’s important to find a balance between the opportunity costs and potential returns and risk to achieve your business goals.

Can’t Be Used to Compare Projects of Different Sizes

The NPV formula tells you how many dollars a project could generate without considering the project size and the return on investment. As a result, projects with big initial capital usually produce high NPV, and vice versa—low capital investments have a low net present value. In other words, the NPV calculation depends mostly on the size of the input. For example, a $10 million project will have a higher NPV than a $10,000 investment, even if the $10,000 deal produces higher percentage returns. This makes the NPV method a poor indicator of financial success when capital is scarce.

Can’t Be Used as a Standalone Metric

While the NPV calculation is a useful tool for evaluating the financial viability of a project, it doesn’t provide a complete picture of all relevant aspects. Let’s consider Alpha Corporation again to explain this in more detail. Suppose that the management must choose between Project A and Project B, using NPV as a key financial metric to evaluate the investments. Upon conducting the analyses, Project A has a positive NPV of $100,000, while Project B has a negative NPV of -$10,000. Based on the net present value calculation alone, the obvious winner is Project A.

However, Alpha Corporation moves forward with executing Project B because it expands into a new market and secures a long-term contract, which is of great strategic value for the organization. Improving its competitive position in the market also happens to be in line with the firm’s goals in the next few years. What’s more, Project B involves upgrading some of the manufacturing facilities, which will actually save costs for other business units as well, making an overall positive impact on operations. And with its stable cash flows, it turns out the risk associated with the investment is lower as compared to Project A.

That’s why, before making such an important decision, organizations usually go through a series of questions regarding the overall situation of those investments, such as:

• Is Project A or Project B the most efficient way to spend the money?
• What’s their return on investment?
• Which project has a greater strategic value?
• Is Project B having a lower risk than Project A?
• Which investment better grows the company and its brand as a whole?

These are all relevant concerns that require professionals to combine the NPV with other efficiency metrics, such as the internal rate of return and payback period, along with some non-financial factors.

Overlooking Non-Financial Benefits

Overall, you calculate the net present value to quantify your projections about the financial viability of a project. However, there may be some intangible factors that are worth considering too. Most of the time, the non-financial benefits of an investment are equally valuable. For company Alpha which we discuss in our example, cutting-edge innovation and technology in shipbuilding mean attracting investors and improving business operations. Such a breakthrough technology could also be a competitive advantage and contribute to future growth. These factors aren’t part of the NPV calculation, but they play an important role in the future cash flows of a project and of a company as a whole.

Showcasing NPV Calculation Skills on Your Resume

If you know how to calculate and interpret NPV, you are a step closer to gaining two valuable skills—financial modeling and business valuation. For those of you who have work or internship experience, you could talk about the NPV analysis when describing the daily tasks you’ve tackled at the time. For instance, you could say that you were able to easily compute and compare the NPV of 10 different investments during your internship at Wells Fargo & Company.  This helped your team to make an informed investment decision, and it increased profitability.

Don’t have any prior work experience with NPV calculations? Use your cover letter to showcase any complementary skills you’ve got that are relevant and valuable to the employer. You can highlight that relying on the NPV calculation goes beyond capital budgeting in big corporations. Feel free to talk about your hands-on experience using various financial modeling techniques when your uncle wanted to open up а retail shop. You may say a word or two about the spreadsheets you’ve built to assist in managing the business.

Don’t forget to mention that now that the business is up and running, you occasionally do his retail budgeting and retirement planning, which are all transferable financial modeling and business valuation skills you could use at any firm. And so, providing real-life examples can support the competencies and achievements you emphasize in your cover letter.

Next Steps

Calculating and interpreting NPV is just a small part of what you need to be proficient in financial modeling and business valuation. If you are determined to pursue a career as a financial analyst, investment banker, or investment analyst, you’ll require a lot more than that. You will be expected to know the basics of corporate governance, measures of leverage, capital budgeting techniques, working capital management, and many more.

Are you ready to take the next step toward a career in finance?

Our Corporate Finance course will provide you with many hands-on examples of how companies can operate more effectively. You’ll have a chance to gain valuable insights into fundamental financial techniques and measures from industry experts with a proven track record. With our Investment Analyst Career Track, you can learn at your own pace and have all your achievements certified, so you can include them in your Resume.

From beginners to seasoned professionals, our courses are suitable for learners of all backgrounds. Choose the course that fits your career goals and start learning today!

FAQs