P-value for Negative T-score on right-tailed.
Hi,
in this example attached in this excel file from the courses, the H0 < 40% and the H1 >40%.
this is a right-tailed since H1 is in the right side, and the T-score calculated is -0.53.
I'm just wondering why the p-value is equal to 0.304

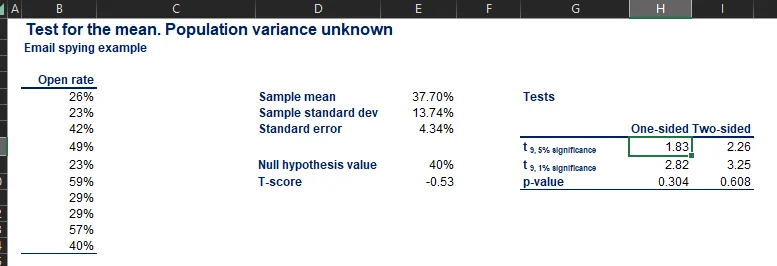
using the online p-value calculator is equal to 0.6955.
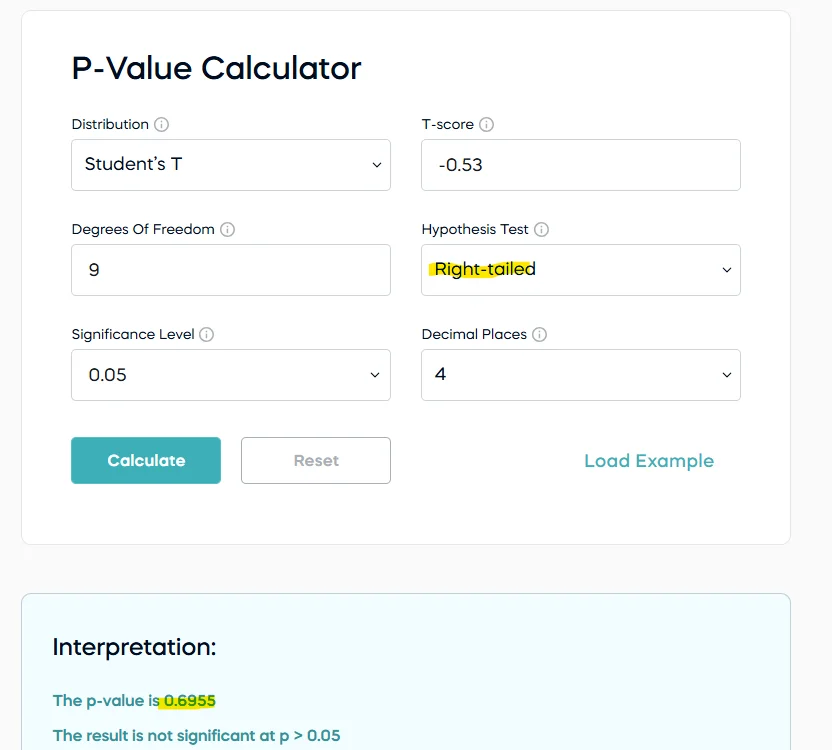
the P-value is corrected only once i change the hypothesis test to left-tailed.
can anyone explain to me why it's only correct with left-tailed ?
1 answers ( 0 marked as helpful)
Hi Haithem!
Thanks for reaching out!
You're correct that the test is right-sided because the alternative hypothesis states that the email open rate is more than 40% (meaning the direction is to the right from the null hypothesis value). This is a great observation you just made.
However, in this case, regardless of whether we calculate the p-value assuming a left-sided or right-sided test, we would still fail to reject the null hypothesis. The null hypothesis remains valid under either assumption.
That said, your observation is absolutely valid. It’s critical to correctly identify the test direction to ensure proper interpretation and alignment with the hypothesis.
Thank you for pointing this out!
Best,
Ivan
Thanks for reaching out!
You're correct that the test is right-sided because the alternative hypothesis states that the email open rate is more than 40% (meaning the direction is to the right from the null hypothesis value). This is a great observation you just made.
However, in this case, regardless of whether we calculate the p-value assuming a left-sided or right-sided test, we would still fail to reject the null hypothesis. The null hypothesis remains valid under either assumption.
That said, your observation is absolutely valid. It’s critical to correctly identify the test direction to ensure proper interpretation and alignment with the hypothesis.
Thank you for pointing this out!
Best,
Ivan